Download free pdf of Linear Equations in One Variable Worksheets for Class 8 with answers. Students can easily get all the Class 8 Maths Worksheets and Exercises in Free Printable ( PDF Format) as per the latest syllabus and examination pattern in CBSE and NCERT pattern. Below you will find all the Linear Equation in One Variable related sums that are asked in 8th Grade Mathematics Exams across Indian Schools.
Linear Equations in One Variable Worksheets for Class 8 in PDF Format
- Class 8 Maths Linear Equation in One Variable Worksheet (3)
- Class 8 Maths Linear Equation in One Variable Worksheet (2)
- Class 8 Maths Linear Equation in One Variable Worksheet (1)
- Linear Equations in One Variable Class 8 Practice Questions
- Linear Equations in One Variable Class 8 Maths Worksheets
- Linear Equations in One Variable Class 8 Worksheet with Answers
Class 8 Chapter 2 Linear Equations Worksheets with answers
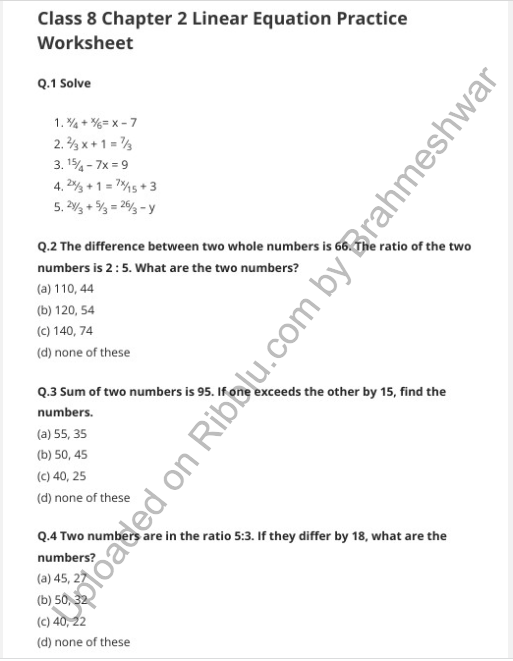
Q.1 Solve
- x⁄4 + x⁄6= x – 7
- 2⁄3 x + 1 = 7⁄3
- 15⁄4 – 7x = 9
- 2x⁄3 + 1 = 7x⁄15 + 3
- 2y⁄3 + 5⁄3 = 26⁄3 – y
Answers:
(1) x/4 + x/6 = x – 7
To solve this equation, let’s first find a common denominator, which is 12 in this case:
(3x + 2x)/12 = 12x – 84
Now, combine the fractions on the left side:
5x/12 = 12x – 84
Next, multiply both sides by 12 to isolate x:
5x = 144x – 1008
Now, subtract 144x from both sides:
5x – 144x = -1008
-139x = -1008
Finally, divide both sides by -139 to find the value of x:
x = -1008 / -139
x ≈ 7.26 (rounded to two decimal places)
(2) 2/3 x + 1 = 7/3
To solve this equation, first subtract 1 from both sides:
2/3 x = 7/3 – 1
2/3 x = 7/3 – 3/3
2/3 x = 4/3
Now, multiply both sides by 3/2 to isolate x:
(2/3 x) * (3/2) = (4/3) * (3/2)
x = (4/3) * (3/2)
x = 2
(3) 15/4 – 7x = 9
To solve this equation, first subtract 15/4 from both sides:
-7x = 9 – 15/4
Now, find a common denominator for 9 and 15/4, which is 4:
-7x = (36/4) – (15/4)
-7x = (36 – 15)/4
-7x = 21/4
Now, divide both sides by -7 to isolate x:
x = (21/4) / -7
x = (21/4) * (-1/7)
x = -3/4
(4) 2x/3 + 1 = 7x/15 + 3
To solve this equation, first subtract 1 from both sides:
2x/3 = 7x/15 + 3 – 1
2x/3 = 7x/15 + 2
Now, find a common denominator for 7x/15 and 2, which is 15:
2x/3 = (7x + 30)/15
Next, multiply both sides by 3 to isolate x:
2x = (7x + 30)/15 * 3
2x = (7x + 30)/5
Now, cross-multiply:
10x = 7x + 30
Subtract 7x from both sides:
10x – 7x = 7x – 7x + 30
3x = 30
Finally, divide both sides by 3 to find the value of x:
x = 30 / 3
x = 10
(5) 2y/3 + 5/3 = 26/3 – y
To solve this equation, first add y to both sides:
2y/3 + y + 5/3 = 26/3
Now, combine like terms on the left side:
(2y + 3y)/3 + 5/3 = 26/3
(5y + 5)/3 = 26/3
Next, subtract 5/3 from both sides:
(5y + 5)/3 – 5/3 = 26/3 – 5/3
(5y + 5 – 5)/3 = 21/3
(5y)/3 = 21/3
Now, multiply both sides by 3 to isolate 5y:
5y = 21
Finally, divide both sides by 5 to find the value of y:
y = 21/5
Q.2 The difference between two whole numbers is 66. The ratio of the two numbers is 2 : 5. What are the two numbers?
(a) 110, 44
(b) 120, 54
(c) 140, 74
(d) none of these
Answer:
Let’s call the two numbers 2x and 5x, where x is a positive whole number.
According to the problem, the difference between these two numbers is 66:
5x – 2x = 66
Now, simplify the equation:
3x = 66
To find the value of x, divide both sides by 3:
x = 66 / 3
x = 22
So, one number is 2x = 2 * 22 = 44, and the other number is 5x = 5 * 22 = 110.
The two numbers are 44 and 110.
Q.3 Sum of two numbers is 95. If one exceeds the other by 15, find the numbers.
(a) 55, 35
(b) 50, 45
(c) 40, 25
(d) none of these
Answer:
Let’s call the two numbers x and y.
According to the problem, the sum of the two numbers is 95:
x + y = 95
It is also given that one number exceeds the other by 15, which can be expressed as:
x = y + 15
Now, we can substitute the value of x from the second equation into the first equation:
(y + 15) + y = 95
Combine like terms:
2y + 15 = 95
Subtract 15 from both sides:
2y = 95 – 15
2y = 80
Now, divide both sides by 2 to find the value of y:
y = 80 / 2
y = 40
Now that we have the value of y, we can find x using the second equation:
x = y + 15 x = 40 + 15 x = 55
So, the two numbers are 55 and 40.
Q.4 Two numbers are in the ratio 5:3. If they differ by 18, what are the numbers?
(a) 45, 27
(b) 50, 32
(c) 40, 22
(d) none of these
Answer:
Let’s call the two numbers 5x and 3x, where x is a positive whole number.
According to the problem, the difference between these two numbers is 18:
5x – 3x = 18
Now, simplify the equation:
2x = 18
To find the value of x, divide both sides by 2:
x = 18 / 2
x = 9
Now that we have the value of x, we can find the two numbers:
First number = 5x = 5 * 9 = 45 Second number = 3x = 3 * 9 = 27
So, the two numbers are 45 and 27, which matches option (a).
Q. 5 Three consecutive integers add up to 51. What are these integers?
(a) 117, 121, 125
(b) 110, 121, 132
(c) 110, 99, 154
(d) none of these
Answer :
Let’s call the three consecutive integers x, x + 1, and x + 2.
According to the problem, their sum is 51:
x + (x + 1) + (x + 2) = 51
Now, combine like terms:
3x + 3 = 51
Subtract 3 from both sides:
3x = 51 – 3
3x = 48
Now, divide both sides by 3 to find the value of x:
x = 48 / 3
x = 16
So, the three consecutive integers are 16, 17, and 18, which matches option (b)
Q.6 Sum of two numbers is 95. If one exceeds the other by 15, find the numbers.
Answer:
Let’s call the two numbers x and y, where x is greater than y.
According to the problem, the sum of the two numbers is 95:
x + y = 95
Also, it’s given that one number exceeds the other by 15:
x = y + 15
Now, we can substitute the value of x from the second equation into the first equation:
(y + 15) + y = 95
Combine like terms:
2y + 15 = 95
Subtract 15 from both sides:
2y = 95 – 15
2y = 80
Now, divide both sides by 2 to find the value of y:
y = 80 / 2
y = 40
Now that we have the value of y, we can find the value of x using the second equation:
x = y + 15 x = 40 + 15 x = 55
So, the two numbers are 55 and 40.
Q.7 Two numbers are in the ratio 5:3. If they differ by 18, what are the numbers?
Answer: The answer is 45 and 27.
Q.8 Three consecutive integers add up to 51. What are these integers?
Answer : The answer is 16, 17, and 18.
Q.9 The sum of three consecutive multiples of 8 is 888. Find the multiples.
Answer:
Let’s call the first multiple of 8 as “x.”
So, the three consecutive multiples of 8 are:
- x
- x + 8 (the next multiple)
- x + 16 (the next multiple after that)
According to the problem, their sum is 888:
x + (x + 8) + (x + 16) = 888
Now, combine like terms:
3x + 24 = 888
Subtract 24 from both sides:
3x = 888 – 24
3x = 864
Now, divide both sides by 3 to find the value of x:
x = 864 / 3
x = 288
So, the three consecutive multiples of 8 are 288, 296, and 304.
Q.10 The ages of Rahul and Haroon are in the ratio of 5:7. Four years from now sum of their ages will be 56 years. Find their present age.
Answer :
Let the present age of Rahul be 5x years and the present age of Haroon be 7x years.
According to the problem, four years from now, the sum of their ages will be 56 years:
(5x + 4) + (7x + 4) = 56
Now, combine like terms:
12x + 8 = 56
Subtract 8 from both sides:
12x = 56 – 8
12x = 48
Now, divide both sides by 12 to find the value of x:
x = 48 / 12
x = 4
Now that we have the value of x, we can find their present ages:
Rahul’s age = 5x = 5 * 4 = 20 years Haroon’s age = 7x = 7 * 4 = 28 years
So, Rahul is 20 years old, and Haroon is 28 years old.
Q.11 Baichung’s father is 26 years younger than Baichung’s grandfather and 29 years older than Baichung. The sum of their ages is 135. Find their ages
Answer:
Let’s denote:
- Baichung’s age as “B”
- Baichung’s father’s age as “F”
- Baichung’s grandfather’s age as “G”
According to the information given, we can create two equations:
- Baichung’s father is 26 years younger than Baichung’s grandfather: F = G – 26
- Baichung’s father is 29 years older than Baichung: F = B + 29
We’re also given that the sum of their ages is 135: B + F + G = 135
Now, we have a system of three equations. Let’s solve it:
From equation (1), we can express G in terms of F: G = F + 26
Now, we can substitute this into equation (3): B + F + (F + 26) = 135
Combine like terms: 2F + 26 + B = 135
Subtract 26 from both sides: 2F + B = 135 – 26 2F + B = 109
Now, we can use equation (2) to express B in terms of F: B = F – 29
Substitute this into the equation above: 2F + (F – 29) = 109
Combine like terms: 3F – 29 = 109
Add 29 to both sides: 3F = 109 + 29 3F = 138
Now, divide by 3 to find the value of F (Baichung’s father’s age): F = 138 / 3 F = 46
Now that we have the age of Baichung’s father, we can find B and G using the earlier equations:
From equation (2): B = F + 29 B = 46 + 29 B = 75
From equation (1): G = F + 26 G = 46 + 26 G = 72
So, Baichung is 75 years old, his father is 46 years old, and his grandfather is 72 years old.
Q.12 Lakshmi is a cashier in a bank. She has notes of denominations of Rs.100, 50 and 10 respectively. The ratio of number of these notes is 2:3:5 respectively. The total cash with Lakshmi is 4,00,000. How many notes of each denomination does she have?
Answer :
Let’s denote:
- The number of Rs.100 notes as “x”
- The number of Rs.50 notes as “y”
- The number of Rs.10 notes as “z”
According to the problem, we have a ratio of 2:3:5 for the number of notes of each denomination:
x:y:z = 2:3:5
We’re also given that the total cash with Lakshmi is Rs. 4,00,000, which can be expressed in terms of the values of these notes:
Total Cash = (Number of Rs.100 notes) * (Value of each Rs.100 note) + (Number of Rs.50 notes) * (Value of each Rs.50 note) + (Number of Rs.10 notes) * (Value of each Rs.10 note)
400000 = (2x) * 100 + (3y) * 50 + (5z) * 10
Now, simplify this equation:
400000 = 200x + 150y + 50z
We can simplify this equation further by dividing everything by 50 to make it easier to work with:
8000 = 4x + 3y + z
Now, we have a system of equations:
- x:y:z = 2:3:5
- 4x + 3y + z = 8000
We can solve this system of equations to find the values of x, y, and z.
Let’s start by solving equation (1) for x, y, and z:
x = (2/10) * k (where k is a common multiple of 2, 3, and 5) y = (3/10) * k z = (5/10) * k
Now, we substitute these expressions into equation (2):
4((2/10) * k) + 3((3/10) * k) + ((5/10) * k) = 8000
Now, simplify this equation:
(8/10)k + (9/10)k + (5/10)k = 8000
Combine like terms and convert fractions to decimals:
(8/10 + 9/10 + 5/10)k = 8000
(22/10)k = 8000
Now, solve for k:
k = (8000 * 10) / 22 k = 36363.63 (approximately)
Now that we have the value of k, we can find the values of x, y, and z:
x = (2/10) * 36363.63 ≈ 7272.73 y = (3/10) * 36363.63 ≈ 10909.09 z = (5/10) * 36363.63 ≈ 18181.82
So, Lakshmi has approximately 7272 Rs.100 notes, 10909 Rs.50 notes, and 18182 Rs.10 notes.
Q.13 Lakshmi is a cashier in a bank. She has notes of denominations of Rs.100, 50 and 10 respectively. The ratio of number of these notes is 2:3:5 respectively. The total cash with Lakshmi is 4,00,000. How many notes of each denomination does she have?
Answer :
Let’s denote:
- The number of Rs.100 notes as “2x” (since the ratio is 2)
- The number of Rs.50 notes as “3x” (since the ratio is 3)
- The number of Rs.10 notes as “5x” (since the ratio is 5)
According to the problem, the total cash with Lakshmi is Rs. 4,00,000, which can be expressed in terms of the values of these notes:
Total Cash = (Number of Rs.100 notes) * (Value of each Rs.100 note) + (Number of Rs.50 notes) * (Value of each Rs.50 note) + (Number of Rs.10 notes) * (Value of each Rs.10 note)
400000 = (2x) * 100 + (3x) * 50 + (5x) * 10
Now, simplify this equation:
400000 = 200x + 150x + 50x
Combine like terms:
400000 = 400x
Now, solve for x:
x = 400000 / 400 x = 1000
Now that we have the value of x, we can find the number of each denomination:
Number of Rs.100 notes = 2x = 2 * 1000 = 2000 notes Number of Rs.50 notes = 3x = 3 * 1000 = 3000 notes Number of Rs.10 notes = 5x = 5 * 1000 = 5000 notes
So, Lakshmi has 2000 Rs.100 notes, 3000 Rs.50 notes, and 5000 Rs.10 notes.
Q.14 The organizers in an essay competition decide that winner will get a prize of Rs. 100 and a participation who doesn’t win gets a prize of Rs. 25.The total prize money distributed is Rs. 3,000. Find the number of winners if the total number of participants is 63.
Answers:
Let’s denote:
- The number of winners as “W”
- The number of participants who don’t win as “D” (since they don’t win, they are essentially participants who didn’t win)
According to the problem, the total prize money distributed is Rs. 3,000, which can be expressed in terms of the prizes awarded:
Total Prize Money = (Number of winners) * (Prize for winners) + (Number of participants who don’t win) * (Prize for participants who don’t win)
3000 = (W) * 100 + (D) * 25
Now, we know that the total number of participants is 63:
Total Participants = Winners (W) + Participants Who Don’t Win (D)
63 = W + D
We have a system of two equations:
- 3000 = 100W + 25D
- 63 = W + D
Let’s solve this system of equations to find the number of winners (W):
From equation (2), we can express D in terms of W: D = 63 – W
Now, substitute this expression into equation (1):
3000 = 100W + 25(63 – W)
Now, simplify the equation:
3000 = 100W + 1575 – 25W
Combine like terms:
3000 = 75W + 1575
Subtract 1575 from both sides:
1425 = 75W
Now, divide by 75 to find the number of winners (W):
W = 1425 / 75 W = 19
So, there are 19 winners in the competition.
Q.15 Sum of the digits of a two digit number is 9. When we interchange the digits the new number is 27 greater than the earlier number. Find the number.
Answer :
Let’s denote:
- The tens digit of the two-digit number as “T”
- The ones digit of the two-digit number as “O”
According to the problem, the sum of the digits is 9:
T + O = 9
When we interchange the digits, the new number is 27 greater than the earlier number:
(10 * O + T) – (10 * T + O) = 27
Now, simplify this equation:
10O + T – 10T – O = 27
Combine like terms:
9O – 9T = 27
Divide both sides by 9 to isolate O – T:
O – T = 27 / 9 O – T = 3
Now, we have a system of two equations:
- T + O = 9
- O – T = 3
Let’s solve this system of equations:
First, add equation (1) and equation (2) to eliminate T:
(T + O) + (O – T) = 9 + 3
Now, simplify:
2O = 12
Divide by 2:
O = 6
Now that we have the value of O, we can find T using equation (1):
T + 6 = 9
Subtract 6 from both sides:
T = 9 – 6 T = 3
So, the tens digit (T) is 3, and the ones digit (O) is 6. Therefore, the two-digit number is 36.
Q.16 One of the digits of a two digit number is three times the other digit. If you interchange the digits and add the resulting number to original number you get 88 as final result. Find the numbers.
Let’s denote:
The tens digit of the two-digit number as “T”
The ones digit of the two-digit number as “O”
According to the problem, one of the digits is three times the other:
T = 3O
When we interchange the digits and add the resulting number to the original number, we get 88:
(10 * O + T) + (10 * T + O) = 88
Now, we can substitute the value of T from equation (1) into equation (2):
(10 * O + 3O) + (10 * 3O + O) = 88
Now, simplify this equation:
13O + 30O + O = 88
Combine like terms:
44O = 88
Divide by 44 to find the value of O:
O = 88 / 44
O = 2
Now that we have the value of O, we can find T using equation (1):
T = 3O
T = 3 * 2
T = 6
So, the tens digit (T) is 6, and the ones digit (O) is 2. Therefore, the two-digit number is 62.
Q.17 Sahoo’s mother’s present age is six times Sahoo’s present age. Five year from now Sahoo’s age will be one-third of his mother’s age. Find their cur-rent age.
Answer :
Let’s denote:
- Sahoo’s present age as “S”
- Sahoo’s mother’s present age as “M”
According to the problem, Sahoo’s mother’s present age is six times Sahoo’s present age:
- M = 6S
Five years from now, Sahoo’s age will be one-third of his mother’s age:
- S + 5 = (1/3)(M + 5)
Now, we can substitute the value of M from equation (1) into equation (2):
S + 5 = (1/3)(6S + 5)
Now, simplify this equation:
3(S + 5) = 6S + 5
Expand and simplify:
3S + 15 = 6S + 5
Subtract 3S from both sides:
15 = 3S + 5
Subtract 5 from both sides:
10 = 3S
Now, divide by 3 to find Sahoo’s present age (S):
S = 10 / 3 S = 3.33 (approximately)
So, Sahoo’s present age is approximately 3.33 years. To find Sahoo’s mother’s present age (M), we can use equation (1):
M = 6S M = 6 * 3.33 M = 20 years (approximately)
Therefore, Sahoo is approximately 3.33 years old, and his mother is approximately 20 years old.
Q.18 Half of a herd of deer are grazing in the field and three fourths of the remaining are playing nearby. The rest 9 are drinking water from the pond. Find the total number of deer in the herd
Answer :
Let’s denote:
- The total number of deer in the herd as “D”
According to the problem, half of the herd is grazing in the field:
- (1/2)D
Three fourths of the remaining herd are playing nearby:
- (3/4)((1/2)D)
The rest 9 are drinking water from the pond:
- 9
The sum of these three parts equals the total number of deer in the herd:
(1/2)D + (3/4)((1/2)D) + 9 = D
Now, we can solve for D:
First, simplify the fractions:
(1/2)D + (3/8)D + 9 = D
Now, subtract (1/2)D and (3/8)D from both sides:
(1/2)D – (1/2)D + (3/8)D – (3/8)D + 9 = D – (1/2)D – (3/8)D
9 = (16/8)D – (11/8)D
Now, combine like terms:
9 = (5/8)D
Now, divide by (5/8) to find D:
D = 9 / (5/8)
To divide by a fraction, multiply by its reciprocal:
D = 9 * (8/5) D = 72/5 D = 14.4
So, the total number of deer in the herd is 14.4, which is not possible for a real-world situation. It appears there is an issue with the problem statement or solution.
Q.19 A man’s age is three times his son’s age. Ten years ago his age was five times his son’s age. Find their current age
Let’s denote:
- The man’s current age as “M”
- His son’s current age as “S”
According to the problem, a man’s age is three times his son’s age:
- M = 3S
Ten years ago, the man’s age was five times his son’s age:
- (M – 10) = 5(S – 10)
Now, we can solve this system of equations to find their current ages:
First, substitute the value of M from equation (1) into equation (2):
(3S – 10) = 5(S – 10)
Now, expand and simplify:
3S – 10 = 5S – 50
Subtract 3S from both sides:
-10 = 2S – 50
Now, add 50 to both sides:
40 = 2S
Divide by 2 to find the son’s current age (S):
S = 40 / 2 S = 20
Now that we know the son’s age, we can find the man’s age using equation (1):
M = 3S M = 3 * 20 M = 60
So, the man is currently 60 years old, and his son is currently 20 years old.
Q.20 If in a rational number denominator is greater than numerator by 8. If you increase the numerator by 17 and decrease the denominator by 1, you get 3/2 as result. Find the number
Answer :
Let’s denote:
- The numerator of the rational number as “N”
- The denominator of the rational number as “D”
According to the problem, the denominator is greater than the numerator by 8:
- D = N + 8
If you increase the numerator by 17 and decrease the denominator by 1, you get 3/2 as the result:
- (N + 17) / (D – 1) = 3/2
Now, we can solve this system of equations to find the rational number:
First, substitute the value of D from equation (1) into equation (2):
(N + 17) / (N + 8 – 1) = 3/2
Simplify the denominator:
(N + 17) / (N + 7) = 3/2
Now, cross-multiply:
2(N + 17) = 3(N + 7)
Expand and simplify:
2N + 34 = 3N + 21
Subtract 2N from both sides:
34 = N + 21
Subtract 21 from both sides:
13 = N
So, the numerator (N) is 13. Now, we can find the denominator (D) using equation (1):
D = N + 8 D = 13 + 8 D = 21
Therefore, the rational number is 13/21.
Q.21 The perimeter of a rectangular swimming pool is 154 m. Its length is 2m more than twice its breadth. What are the length and breadth of the pool?
Answer:
Let’s denote:
- The length of the rectangular swimming pool as “L”
- The breadth of the rectangular swimming pool as “B”
According to the problem, the length is 2 meters more than twice the breadth:
- L = 2B + 2
The perimeter of a rectangle is given by the formula: Perimeter = 2(L + B)
In this case, the perimeter is given as 154 meters:
2(L + B) = 154
Now, we can substitute the value of L from equation (1) into the perimeter equation:
2((2B + 2) + B) = 154
Now, simplify the equation:
2(3B + 2) = 154
Expand:
6B + 4 = 154
Subtract 4 from both sides:
6B = 150
Now, divide by 6 to find the breadth (B):
B = 150 / 6 B = 25
Now that we have the breadth, we can find the length (L) using equation (1):
L = 2B + 2 L = 2 * 25 + 2 L = 50 + 2 L = 52
So, the length of the rectangular swimming pool is 52 meters, and the breadth is 25 meters.
Q.22 Two numbers are in the ratio 8:3. If sum of the numbers is 143, find the numbers
Answer :
Let’s denote:
- The first number as “8x” (since it’s in the ratio of 8)
- The second number as “3x” (since it’s in the ratio of 3)
According to the problem, the sum of the numbers is 143:
- 8x + 3x = 143
Now, combine like terms:
11x = 143
To isolate x, divide both sides by 11:
x = 143 / 11 x = 13
Now that we have the value of x, we can find the two numbers:
First number = 8x = 8 * 13 = 104 Second number = 3x = 3 * 13 = 39
So, the two numbers are 104 and 39.
Q.23 Four-fifths of a number is 10 more than two-thirds of the number. Find the number
Answer :
Let’s denote the number as “N.”
According to the problem, four-fifths of the number is 10 more than two-thirds of the number:
- (4/5)N = (2/3)N + 10
To solve for N, first subtract (2/3)N from both sides:
(4/5)N – (2/3)N = 10
Now, find a common denominator, which is 15:
(12/15)N – (10/15)N = 10
Combine like terms:
(2/15)N = 10
Now, multiply both sides by 15/2 to isolate N:
N = (10 * 15) / 2 N = 150 / 2 N = 75
So, the number is 75.
Q.24 If 10 be added to four times a certain number, the result is 5less than five times the number. Find the number
Answer :
Let’s denote the certain number as “N.”
According to the problem, if 10 is added to four times the number, the result is 5 less than five times the number:
- 4N + 10 = 5N – 5
Now, subtract 4N from both sides:
10 = N – 5
Add 5 to both sides to isolate N:
N = 10 + 5 N = 15
So, the number is 15.