Download free pdf of Chapter 12 Ratio and Proportion Worksheets for Class 6 Maths with answers. Students can easily get all the Class 6 Maths Worksheets and Exercises in Free Printable ( PDF Format) as per the latest syllabus and examination pattern in CBSE and NCERT pattern. Below you will find all the Ratio and Proportion related sums that are asked in 6th Grade Mathematics Exams Across Indian Schools.
Ratio and Proportion Worksheets for Class 6 in PDF Format
- Class 6 Maths Ratio and Proportion Worksheet – 6
- CBSE Class 6 Maths Worksheet Ratio and Proportion – 6
- CBSE Class 6 Maths Worksheet Ratio and Proportion – 5
- CBSE Class 6 Maths Worksheet Ratio and Proportion – 4
- CBSE Class 6 Maths Worksheet Ratio and Proportion – 3
- CBSE Class 6 Maths Worksheet Ratio and Proportion – 2
- CBSE Class 6 Maths Worksheet Ratio and Proportion – 1
- Class 6 Maths Ratio and Proportion Worksheet-5 with Answer
- Class 6 Maths Ratio and Proportion Worksheet-4 with Answer
- Class 6 Maths Ratio and Proportion Worksheet-3 with Answer
- Class 6 Maths Ratio and Proportion Worksheet-2 with Answer
- Class 6 Maths Ratio and Proportion Worksheet-1 with Answer
- Ratio and Proportion CBSE Class 6 Worksheet for Maths
- Ratio and Proportion CBSE Class 6 Maths Worksheet
- Worksheet on Ratio and Proportion for CBSE Class 6 Maths
- Maths Worksheets for CBSE Class 6 Ratio and Proportion
- Ratio and Proportion CBSE Class 6 Maths Worksheet in PDF
- Class 6 Maths Worksheet – Ratio and Proportion
- Class 6 Maths MCQ Worksheet 6 – Ratio and Proportion
- Class 6 Maths MCQ Worksheet 5 – Ratio and Proportion
- Class 6 Maths MCQ Worksheet 4 – Ratio and Proportion
- Class 6 Maths MCQ Worksheet 3 – Ratio and Proportion
- Class 6 Maths MCQ Worksheet 2 – Ratio and Proportion
- Class 6 Maths MCQ Worksheet 1 – Ratio and Proportion
- Ratio and Proportion Class 6 Maths Worksheets
Class 6 Ratio and Proportion Worksheets with answers
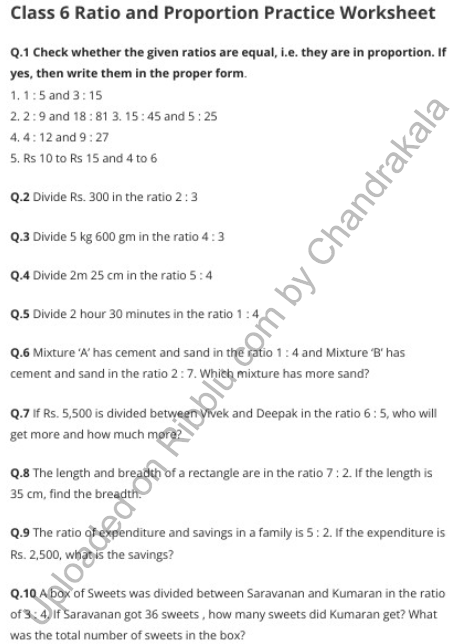
Q.1 Check whether the given ratios are equal, i.e. they are in proportion. If yes, then write them in the proper form.
1. 1 : 5 and 3 : 15
2. 2 : 9 and 18 : 81 3. 15 : 45 and 5 : 25
4. 4 : 12 and 9 : 27
5. Rs 10 to Rs 15 and 4 to 6
Answers:
- Yes, the ratios are equal. Proper form: 1 : 5 (both ratios are equivalent).
- Yes, the ratios are equal. Proper form: 2 : 9 (both ratios are equivalent).
- Yes, the ratios are equal. Proper form: 15 : 45 (both ratios are equivalent).
- Yes, the ratios are equal. Proper form: 4 : 12 (both ratios are equivalent).
- Yes, the ratios are equal. Proper form: Rs 10 to Rs 15 (both ratios are equivalent).
Q.2 Divide Rs. 300 in the ratio 2 : 3
Answer:
Total parts = 2 parts + 3 parts = 5 parts
Each part = Rs. 300 / 5 = Rs. 60
2 parts = 2 * Rs. 60 = Rs. 120
3 parts = 3 * Rs. 60 = Rs. 180
So, the division is Rs. 120 : Rs. 180, or simplified to 2 : 3.
Q.3 Divide 5 kg 600 gm in the ratio 4 : 3
Answer:
Total parts = 4 parts + 3 parts = 7 parts
Each part = 5 kg 600 gm / 7 = 800 gm
4 parts = 4 * 800 gm = 3200 gm = 3.2 kg
3 parts = 3 * 800 gm = 2400 gm = 2.4 kg
So, the division is 3.2 kg : 2.4 kg, or simplified to 8 : 6, which further simplifies to 4 : 3.
Q.4 Divide 2m 25 cm in the ratio 5 : 4
Answer:
Total parts = 5 parts + 4 parts = 9 parts
Each part = (2m 25 cm) / 9 = 25 cm
5 parts = 5 * 25 cm = 125 cm = 1 m 25 cm
4 parts = 4 * 25 cm = 100 cm = 1 m
So, the division is 1 m 25 cm : 1 m, or simplified to 5 : 4.
Q.5 Divide 2 hour 30 minutes in the ratio 1 : 4
Answer:
Total parts = 1 part + 4 parts = 5 parts
Each part = (2 hours 30 minutes) / 5 = 30 minutes
1 part = 30 minutes
4 parts = 4 * 30 minutes = 120 minutes = 2 hours
So, the division is 30 minutes : 2 hours, or simplified to 1 : 4.
Q.6 Mixture ‘A’ has cement and sand in the ratio 1 : 4 and Mixture ‘B’ has cement and sand in the ratio 2 : 7. Which mixture has more sand?
Answer:
To determine which mixture has more sand, we can compare the ratios of sand to the total mixture in both Mixtures ‘A’ and ‘B’.
In Mixture ‘A’:
Sand : Total mixture = 4 : (1 + 4) = 4 : 5
In Mixture ‘B’:
Sand : Total mixture = 7 : (2 + 7) = 7 : 9
Comparing the two ratios, we can see that Mixture ‘B’ has a higher ratio of sand to the total mixture. Therefore, Mixture ‘B’ has more sand.
Q.7 If Rs. 5,500 is divided between Vivek and Deepak in the ratio 6 : 5, who will get more and how much more?
Answer:
To find out who will get more and how much more, we need to calculate the share of each person:
Total amount = Rs. 5,500
Ratio of Vivek’s share to Deepak’s share = 6 : 5
Let’s assume Vivek gets 6x and Deepak gets 5x (where x is a common multiple):
Vivek’s share = 6x
Deepak’s share = 5x
Now, we can set up an equation:
6x + 5x = 5,500
Combining like terms:
11x = 5,500
Now, solve for x:
x = 5,500 / 11
x = 500
So, Vivek’s share = 6x = 6 * 500 = Rs. 3,000
Deepak’s share = 5x = 5 * 500 = Rs. 2,500
Now, we can determine who gets more and how much more:
Vivek gets more.
The difference in their shares = Vivek’s share – Deepak’s share = Rs. 3,000 – Rs. 2,500 = Rs. 500 more.
Q.8 The length and breadth of a rectangle are in the ratio 7 : 2. If the length is 35 cm, find the breadth.
Answer:
Given:
Ratio of length to breadth = 7 : 2
Length = 35 cm
Let’s assume the breadth is 2x (where x is a common multiple):
Length = 7x
Breadth = 2x
We know the length is 35 cm, so:
7x = 35
Now, solve for x:
x = 35 / 7
x = 5
Now that we have the value of x, we can find the breadth:
Breadth = 2x = 2 * 5 = 10 cm
So, the breadth of the rectangle is 10 cm.
Q.9 The ratio of expenditure and savings in a family is 5 : 2. If the expenditure is Rs. 2,500, what is the savings?
Answer:
Given:
Ratio of expenditure to savings = 5 : 2
Expenditure = Rs. 2,500
Let’s assume savings is 2x (where x is a common multiple):
Expenditure = 5x
We know the expenditure is Rs. 2,500, so:
5x = 2,500
Now, solve for x:
x = 2,500 / 5
x = 500
Now that we have the value of x, we can find the savings:
Savings = 2x = 2 * 500 = Rs. 1,000
So, the savings in the family is Rs. 1,000.
Q.10 A box of Sweets was divided between Saravanan and Kumaran in the ratio of 3 : 4. If Saravanan got 36 sweets , how many sweets did Kumaran get? What was the total number of sweets in the box?
Given:
Ratio of sweets divided between Saravanan and Kumaran = 3 : 4
Saravanan got 36 sweets.
Let’s assume Kumaran got 4x sweets (where x is a common multiple):
Saravanan’s share = 3x
Kumaran’s share = 4x
We know that Saravanan got 36 sweets, so:
3x = 36
Now, solve for x:
x = 36 / 3
x = 12
Now that we have the value of x, we can find Kumaran’s share:
Kumaran’s share = 4x = 4 * 12 = 48 sweets
To find the total number of sweets in the box, add their shares:
Total sweets = Saravanan’s share + Kumaran’s share = 36 + 48 = 84 sweets
So, Kumaran got 48 sweets, and the total number of sweets in the box was 84.
Q.11 In a class, there are 20 boys and 40 girls. What is the ratio of the number of boys to the number of girls?
Answer:
Number of boys = 20
Number of girls = 40
The ratio of the number of boys to the number of girls can be written as:
20 : 40
To simplify the ratio, we can divide both numbers by their greatest common divisor (which is 20 in this case):
20 ÷ 20 : 40 ÷ 20
1 : 2
So, the ratio of the number of boys to the number of girls is 1 : 2.
Q.12 Ravi walks 6 km in an hour while Roshan walks 4 km in an hour. What is the ratio of the distance covered by Ravi to the distance covered by Roshan?
Answer:
Distance covered by Ravi = 6 km
Distance covered by Roshan = 4 km
The ratio of the distance covered by Ravi to the distance covered by Roshan is:
6 : 4
To simplify the ratio, we can divide both numbers by their greatest common divisor (which is 2 in this case):
6 ÷ 2 : 4 ÷ 2
3 : 2
So, the ratio of the distance covered by Ravi to the distance covered by Roshan is 3 : 2.
Q.13 Saurabh takes 15 minutes to reach school from his house and Sachin takes one hour to reach school from his house. Find the ratio of the time taken by Saurabh to the time taken by Sachin.
Answer:
Time taken by Saurabh = 15 minutes
Time taken by Sachin = 1 hour = 60 minutes
The ratio of the time taken by Saurabh to the time taken by Sachin is:
15 : 60
To simplify the ratio, we can divide both numbers by their greatest common divisor (which is 15 in this case):
15 ÷ 15 : 60 ÷ 15
1 : 4
So, the ratio of the time taken by Saurabh to the time taken by Sachin is 1 : 4.
Q.14 Cost of a toffee is 50 paise and cost of a chocolate is Rs 10. Find the ratio of the cost of a toffee to the cost of a chocolate.
Answer:
Cost of a toffee = 50 paise (0.50 rupees)
Cost of a chocolate = Rs 10
To find the ratio of the cost of a toffee to the cost of a chocolate, we need to express both costs in the same units, either paise or rupees. Let’s express both in paise (since 1 rupee = 100 paise):
Cost of a toffee = 50 paise
Cost of a chocolate = 10 * 100 paise = 1000 paise
Now, we can find the ratio:
50 : 1000
To simplify the ratio, we can divide both numbers by their greatest common divisor (which is 50 in this case):
50 ÷ 50 : 1000 ÷ 50
1 : 20
So, the ratio of the cost of a toffee to the cost of a chocolate is 1 : 20.
Q.15 In a school, there were 73 holidays in one year. What is the ratio of the number of holidays to the number of days in one year?
Answer:
Number of holidays = 73
Total number of days in a year = 365 (assuming it’s a non-leap year)
Ratio of holidays to days in a year = 73 : 365
Simplified ratio = 1 : 5
Q.16 Length and breadth of a rectangular field are 50 m and 15 m respectively. Find the ratio of the length to the breadth of the field.
Answer:
Length = 50 m
Breadth = 15 m
Ratio of length to breadth = 50 m : 15 m
Simplified ratio = 10 : 3
Q.17 Neelam’s annual income is Rs. 288000. Her annual savings amount to Rs. 36000. Find the ratio of her savings to her expenditure.
Answer:
Savings = Rs. 36,000
Annual income = Rs. 2,88,000
Expenditure = Annual income – Savings = Rs. 2,88,000 – Rs. 36,000 = Rs. 2,52,000
Ratio of savings to expenditure = Rs. 36,000 : Rs. 2,52,000
Simplified ratio = 1 : 7
Q.18 Mathematics textbook for Class VI has 320 pages. The chapter ‘symmetry’ runs from page 261 to page 272. Find the ratio of the number of pages of this chapter to the total number of pages of the book.
Answer:
Number of pages in the chapter ‘symmetry’ = 272 – 261 + 1 (inclusive) = 12 pages
Total number of pages in the book = 320 pages
Ratio of chapter pages to total pages = 12 : 320
Simplified ratio = 3 : 80
Q.19 On a shelf, books with green cover and that with brown cover are in the ratio 2:3. If there are 18 books with green cover, then the number of books with brown cover.
Answer:
Given: Ratio of green cover books to brown cover books = 2 : 3
Number of green cover books = 18
Let the number of brown cover books be 3x (to maintain the ratio)
2 : 3 = 18 : 3x (cross-multiplication)
2 * 3x = 18 * 3
6x = 54
x = 54 / 6 = 9
Number of brown cover books = 3x = 3 * 9 = 27 books
Q.20 The length and breadth of a steel tape are 10m and 2.4cm, respectively. Find the ratio of the length to the breadth.
Answer:
Length = 10 m
Breadth = 2.4 cm (0.024 m)
Ratio of length to breadth = 10 m : 0.024 m
Simplified ratio = 125 : 3
Q.21 Income of Rahim is Rs 12000 per month and that of Ami is Rs 191520 per annum. If the monthly expenditure of each of them is Rs 9960 per month find the ratio of their savings.
Answer:
Rahim’s annual income = Rs. 12,000 * 12 months = Rs. 1,44,000
Ami’s annual income = Rs. 1,91,520
Monthly expenditure of each = Rs. 9,960
Rahim’s annual savings = Annual income – Annual expenditure = Rs. 1,44,000 – Rs. 9,960 * 12 = Rs. 1,44,000 – Rs. 1,19,520 = Rs. 24,480
Ratio of Rahim’s savings to Ami’s savings = Rs. 24,480 : Rs. 0 (since Ami’s savings are not mentioned)
Note: The ratio is undefined because Ami’s savings are not given.
Q.21 20 tons of iron costs Rs 600000. Find the cost of 560 kg of iron.
Answer:
Cost of 20 tons of iron = Rs. 6,00,000
Cost of 1 ton of iron = Rs. 6,00,000 / 20 = Rs. 30,000
Cost of 560 kg (0.56 tons) of iron = Rs. 30,000 * 0.56 = Rs. 16,800
Q.22 Find the ratio of the number of sides of a square to the number of edges of a cube.
Answer:
A square has 4 sides.
A cube has 12 edges.
Ratio of sides of a square to edges of a cube = 4 : 12
Simplified ratio = 1 : 3
Q.23 The cost of a notebook is Rs. 20 and the cost of a pen is Rs. 15. What is the ratio between the cost of a notebook and the cost of a pen?
Answer:
Cost of a notebook = Rs. 20
Cost of a pen = Rs. 15
Ratio of cost of a notebook to cost of a pen = Rs. 20 : Rs. 15
Simplified ratio = 4 : 3